How to calculate Future Value of your monthly Investments
POSTED BY ON May 23, 2010 COMMENTS (59)
Lets say you want to invest Rs 2,000 per month for 10 yrs and then want to leave it for next 20 yrs to grow . How will you calculate it ? Do you know ?
Today we will see this basic calculation and learn how to find out the amount you can generate .
We have to understand that there are two phases to this calculation. First is Payment Phase, which is total time when you will pay money from your pocket , example 10 yrs .
Next phase is Investment Phase, This is total time frame you are invested in something product. Example 30 yrs, So in this case Phase 2 – Phase 1 = 20 yrs , which is the time when you let your money grow .
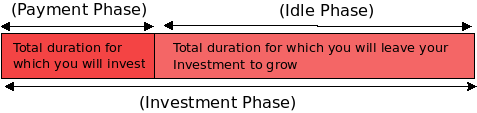
What it means is that your money will grow in two phases, First is the payment phase when you are investing money from your pocket, at the end of the payment phase , you will build a corpus which you can call as “Payment phase Corpus”, Now after this you stop payment any amount from your pocket and just let this “Payment phase coupus” grow year by year in some product till your target date.
So as per our earlier example, You may want to pay for 10 yrs (payment phase) and then let it grow for next 20 yrs and at the end of 30 yrs (Total Investment phase) you will build the “Investment Corpus” .
We will see an example calculation below . Assumptions are
Ajay wants to invest Rs 4,000 per month for 10 yrs and expects a return of 12% yearly (Payment Tenure) . After 10 yrs of investing from his pocket he then wants to leave that investment to grow in Equity (see suggestions for equity funds) and expects it to grow by same 12% return.
His total Investment tenure is 30 yrs. (Video tutorial for calculations)
Calculations
Payment Phase : Our first task here is to calculate the Corpus generated after Payment tenure first . So as per example, Ajay wants to invest Rs 4,000 per month for 10 yrs (120 payments) @12% return expectation . The forumla you have to apply is called Future Value forumla or annuity due (payment in the start of the period) . The forumla is :
FV = A x (1+R) x (((1+R) ^ n) – 1)/R
where
A = Investment per month : This is the amount invested per month , In our example its 4,000 per month
R = Rate of Interest per month (yearly interest/12) . This is monthly return you expect , If you expect the return to be 12% per year , then per month return will be 1% (compounded monthly) , hence R = 1% or 0.01
n = This is total number of payments , so multiply 12 by the number of years , so if your duration is 10 yrs ,then n = 12X10 = 120
As per the formula
FV = 4000 x (1+.01) x (((1+.01) ^ 120) – 1)/.01
= 9,29,356
So we have found that the total corpus generated after 10 yrs of payment tenure is Rs 9,29,356 . First step is completed .
Investment Phase : Here , we are going to calculate the final value of the corpus at the end of Investment phase , so as per step 1 , we have Rs 9,29,356 at the end of 10 yrs , which we will call as Payment phase Corpus (PPC) . Now this amount will be lying in the investment for growth . We just have to apply compound interest formula now which is:
Final Corpus = PPC x (1+R) ^ n
where
PPC = Payment Phase Corpus , we have calculated it above and its value is 9,29,356
R = Rate of return expected for the rest of the period , we have expected it to be 12% or 0.12
n = this is the number of years we are letting the money grow after Payment phase . In our example it was 20 yrs, because total investment tenure was 30 yrs, out of which first 10 yrs was payment tenure .
Applying the formula we get
Final Corpus = 9,29,356 x (1+ 0.12) ^ 20
= 89,64,840
So the final amount you can generate by investing 4,000 per month for 10 yrs and then leaving it to grow for next 20 yrs @12% is 89.64 lacs.
Calculator
You can use the calculator Below to find out your Corpus (Look at more calculators)
Comments please , Did you find this whole calculations very tough to understand ? Suggestions ?
Hi,
Can someone help me understand what the annual rate of return would be in the below mentioned scenario and how to calculate it:
If you are paying INR 5,670/month for 10 years and after another 10 years i.e. total term is 20 years you get back INR 11,00,000 (11 Lakh).
Did you try to use XIRR method on this ?
I mean, what will the formula be if a person puts 4000 rs in a RFD account every month for 10 years and earns, not simple interest but compound interest of 12% pa, the conversion period of which is say, a quarter?
Hi Rahul
It will be approx 12000*((1.03)^40 – 1) /.03
But he is putting 4000 every month. That makes it 48000 in a year. Why are we taking 12000?
We have to look at things from quarter basis as its compounded quarterly.
So amount put per quarter is 12000 , tenure is 4 times , and interst rate is divided by 4
Thank you so much. Understood the concept
Great post. Just one question. What will be the formula if* compounding is also done, say half yearly or quarterly? Rest of the situation remains the same ie. monthly payments of 4000, interest @ 12% pa., years= 10
What do you mean by “compounding is done” ?
Manish, during the payment phase, we are assuming that payments will be compounded monthly, but realistically speaking, no bank in India does monthly compounding, the minimum you can expect is quarterly.
Taking the same example, if monthly payment is Rs. 4000, then quarterly payment is Rs. 12,000. Using the same formula and assuming quarterly compounding, the FV of the corpus (at payment phase) is Rs. 5,88,032 (3% quarterly interest, 30 quarters). The final corpus works out to Rs. 62,57,186, assuming quarterly compounding again.
Let me know if my calculations are correct.
Yes, practically speaking thats correct . But what is the compounding period in mutual funds ? We have considered it to be mutual funds here .
Hi Manish,
I am planning to start investing 2k PM in MF’s.Do we have the chance of investing more money when the market is down keeping 2k investment as usual.
Thanks,
Sandeep
Why not, you can invest more money manually whenever you want !
Sir,
Have joined job recently. Have sister’s marriage down the line in around 2019(7years). Can save and allocate 12000 every month. How should i go about to get maximum returns. Cant take too much risk as objective is marriage.
please suggest options.
regards,
Raj
Raj
I think you can invest 6,000 per month in Balanced funds and rest in 5 yrs FD and Debt Funds , That should keep a balance between returns and risk
Hi Manish
I just came across this website and find it very informative. I have a question for you. Can I use the method articulated above to calculate returns on my VPF contribution. My employer allows me to put aside 88% of my basic for my VPF (Voluntary Provident Fund) which gets compounded at 8.5% PA. The deduction happens on a monthly basis from my payroll but my understanding is that the interest calculation happens once in a year.Can you tell me how I can calculate the return on my VPF where I set aside 9000 rupees per month? How is this same/different from calculating the return on my monthly SIP Mutual Fund investments.
Ananth
The simple way is this , you contribute 1.08 lacs (9 x 12) per year and assuming its getting compounded at 8.5% , you can now use annuity formula here .
So it would be
A * (1+r) * [{(1+r)^t} – 1 ]/r
Where
A = 1.08 lacs
r = 8.5% or .085
t = years
Manish
Manish – I have a quickie – I have SIPs in large cap oriented HDFC Top 200 and also Reliance Banking fund. I infact have these SIPs on different dates – so if I want to invest 10k in a fund in a month I do it as 5 different SIPs for 2000 each so I can cost average even better ;-). On the days that markets fall 200+ points (roughly, more than 1 %) I pump in a little extra cash into these funds as well by 3 PM – well anticipating the markets would not recover greatly by EOD!
I have read with numbers that the DIP has not beat a monthly SIP over any duration but I am thoroughly convinced that 5 SIPs a month is cost effective even if it only a few rupees or decimals of units.
Now for a regular monthly investment (say 5th of every month) the annualized returns can be calculated. How can I do the same for investments made in a fund some 80 – 90 times as year (5 SIPs per month * 12 plus ~ 30 days that I invest when markets fall more). Any tracker to that effect would be helpful!
Anand
You can use something called XIRR to calculate the returns done irregularly : http://jagoinvestor.dev.diginnovators.site/2009/08/what-is-irr-and-xirr-and-how-to.html
Manish
Hello Manish,
Once again a great article. It will be really appreciated, if along with it you provide some suggestions about where to invest with the fund names or scheme names so that the people who do not have much knowledge about these things get a idea about in which funds and where to invest their money. ( Also mention that the risk factor is involved and it is only a suggestion and people need to invest on their own risk )
But it will be more helpful
Thanks for the article, really helpful
Pradeep
Within the article itself , I have given the link to suggestion
http://jagoinvestor.dev.diginnovators.site/2009/08/list-of-best-equity-diversified-mutual.html
Manish
Hi Manish,
Thanks but my suggestion was about not just the mutual funds, but a whole portfolio. As there are many people out there who have less knowledge of all these things.
Ofcourse the website is itself a lot whole new learning experience for many people, which itself is a great work by you.
Thanks a Lot Manish, I have found your site to be a enabler. Infact i need to credit you for a lot of planning that i have been able to accomplish on my own. I have juggled my insurance portfolio where i dumped a Bad Endowment Policy and taken the I-Term. Have used the proceeds and the monthly savings to invest in some good SIP. Additionally have made some adjustments in my outlook and investment plan.
I have been searching for this particular formulae for some time now (was lazy to research 🙂 _) . Extremly helpful. Would you be able to help me understand if there is a concept called as the SIP rate of return. If so can you help in the calcuation. Cheers Keep up the Great work.
Prashanth
Thanks 🙂 . You can use the same formula for SIP and find out the R part . So the way we calculate the SIP is
Final value = A * ((1+r)^n – 1)/.n [Considering payment at the end of the period and not starting) , restructuring the same , we get that
r = {[(A * n) + 1 ]^(1/n)}-1
However the formula i gave in the article was for SIP done in the start of the period and finding a closed form (a proper formula) for r will not be easy , in which case you can either find r using numerical methods or reverse engineer the final value of SIP on different values of r and find appropriate R .
Manish
Manish,
Taking the example mentioned about ajay’s 4k per month invested for 10 yrs and leaving it to grow for 20yrs . Your calculator gave the following results.
Total Corpus : 3759763
Payment Corpus : 929356
Total amount Paid : 480000
Which is correct the figures mentioned in the article or the calculator ?
Hi Manish,
Good Article. But in your calculation of the total amount, you havent factored in inflation! Even if I take inflation at 6%p.a., the final total of 89,64,840 will actually be worth Rs 15.5 lakhs approx today. Kindly shed some light on this. Thanks!
Karan
Inflation has nothing to do with what we have done in this article , its only calculating what will be the value in numbers , Wheather the amount generated at end will be sufficient for some goal is a different issue . Dont you think so ?
Manish
Hi Manish,
You really doing greate and really doing “JAGO” investors!!!!
Thanks for the post.
thanks Prashant
Keep coming
Manish
I think i wanna see more of such posts since they are something that you don’t see these kind of posts much anywhere.
Cannot express in words how important these calculators are. They can make your aimless life much more meaningful and help plan for the future.
It would be really disappointing not to see more of such posts from you, the owner of one of the best blogs in india or maybe in the whole world.
Keep on posting on topics that are based on everyday life.
KSL
KSL
great to hear from you . I would like to write more posts like these , can you give more suggestions on what else you would like to read on , what are other day to day things which a person wants to plan for ?
Manish
write about smart loans please.
Hi , very nice article.Keep up the good work.Just one question , is it safe to assume that i would be able to earn 15 % for next 30 years ? And has it ever happened from nifty or sensex inception it has given return in negative from medium term to long term basis i.e. from 5-10 year basis ? Thanks in advance.
Saurabh
Sexsex till now has given more than 15% return in last 30 yrs of its existence . However there can be sub-periods where it has failed to give that kind of return . that was a different time and coming few decades will be differnet , it will have more uncertainity . So it would be better to assume around 12% return without doing anything from your side (buy and hold with 2-3 yrs review) , or 15% in long term only if you assume that you will do review every year and portfolio rebalancing every 6 month or yrs .
Manish
Very informative article.
Atul
Thanks
Manish
Manish!!
Good one!will be very usefull for basic investors…also helps to find X amount at the end of specific years time then how much to start investing today…….
TS GNANADEV
Yes , I will put another calculator to find that . Should be easy one
Manish
Actually I would like to it will be better if i stay invested in a single equity fund for 30 years… or If i swicth from 1 equity funds to next equity fund after 10 years and keep the amount invested in first one for next 20 years…
Manish
How does that matter , what we have to look at is returns , which ever situation gives better return will make you most of the money .
one more point is , we are not talking about products in this article at all , we are all talking about returns , so if payment phase is 10 yrs and return expected is 12% , that means you are gettting 12% through out the term , thats all , how you get 12% , calculator does not know it . you can shift your money from one place to another any number of times .
Manish
Hi Manish,
Had a basic question, may be a silly one too.
Everybody tells you invest in Mutual Funds, My question is How is the NAV calculated for MF ?
The question arises because, I had invested in Reliance Natural Resources Fund in Jan 2008 when NAV was 10.21, Current NAV is 9.21. Its top 5 holding are Reliance Inds. 7.92, ONGC 5.25, Hind. Petrol 3.79, Tata Steel 3.74, Triveni 3.15.
The Markets have zoomed away and also the top 5 holdings have zoomed but their NAV still remains the same or shold say its negative.
If you can shed some light that would be appreciated.
Regards
Nipi
Nipi
Value or purchase price of a share of stock in a mutual fund. NAV is calculated each day by taking the closing market value of all securities owned plus all other assets such as cash, subtracting all liabilities, then dividing the result (total net assets) by the total number of shares outstanding.
Regarding your question , I have not looked into it in detial , but i can make this comment, You just checked top 5 holdings of the MF , what about the value of rest of the portfolio , it might happen that these 5 shares went up , but rest of other shares went down and the whole value of NAV came down ? Check that .
Manish
Manish,
As most of the people wont be comfortable with leaving the huge amount like 10 lakh,20 lakh in Stock Markets as it is volatile after reaching the such huge figures they might move the amount to safer deposits for portfolio rebalancing so the idle phase calculation may not be usefull for all.
Krishna
There is no restriction on the % value you can put in the calculator , the calculator doesnt know where you are investing , so if one does not want to invest money in pure stock markets , one can move to debt and put 7-9% in the calculators 🙂 . What do you say ?
Manish
Manish
🙂 I agree with you calculator doesn’t know anything.
Manish
If you move the money from equity to debt for rebalancing it will hit on the final corpus amount as the compounding effect isnt there. So in that case what we need to do should we stay invested till we are close to target amount regardless of the how much money is in stock market?.What is ur view?
Krishna
No , Rebalancing will not always hit you . It will hit you only when markets are going up and up , because then you will loose out from shifting to debt . But does stock market go up and up always ? NO !!
Rebalancing means keep shifting money in equity and debt periodically to make sure your asset allocation ratio is constant .
A guy who wants to invest money for next 20 years and then shift it all to debt for next 20 yrs can be a guy who is 30 yrs old guy who wants to invest aggresively in Equity for 20 yrs and once he is close to retirement he wants to shift to debt side no matter what happens in equity .
As you agreed that calculator does not know what you want to do , it just needs numbers , you can always put numbers for what you want .
Manish
Manish Ji
Just to verify about the outcome of the calculation:
I invest 2000/-p.m. for 10 years.
Return is 10%
Investment(idle phase) 10 years
Expected return: 10 years
As per calculation it is like this:
Total Corpus : 1071485
Payment Corpus : 413104
Total amount Paid : 240000
Hope i have done the maths right, Sir.
Best regards
Das
Das
Yes looks good 🙂 . But where will you invest ? Which product ? which thing can give you 10% return , any thoughts ?
Manish
Invest in fairly managed Index Fund. Ex: Benchmark CNX 500 Fund… i have just started investing in VIP mode. Expected CAGR is about 15% in next 15 years 🙂
Ravii
Yea .. I expect it to be 🙂
Manish
I have a question …….. Suppose I invest 4K thru SIP in a equity fund for next 30 years.
Now which one of below case will genrate more money.
Case I
If i stay invested in a single fund lets say Reliance Growth for next 30 years….and let it grow for nex 10 years……….
Case II
If i stay invested in a single fund lets say Reliance Growth for next 10 years(2011-2020) .. and let it grow for next 20 years(2021-2040)…. and start investing in another fund lets say HDFC top 200(after 10 years from 2021-2010) and let it grow for next 10 years……..and start investing in another fund lets say SBI Magnum Contra(after 10 years from 2031-20400) and let it grow for next 10 years……..will genrate me more money……..
Which one of the above case will help me to genrate more money ?.. I suppose i have not made the question complex
Manish
You should not take cases where you have taken different mutual funds names , you should rather talk about the asset class only like Equity and Debt . the product behind it can be anything
Give me an example use the words only Equity and debt and we can plan for it .
Manish
Nice! thanks.
Nicely explained about systemic investments return, calculators are good to use and for long term investment valuations idea one can use.
Thanks
Khalid
thanks for the comment , Do you feel calculators like these and the process will help people to plan for their future ? Some readers find it too complex even if its not .
Manish
Manish, Excellent article. However I am totally confused on the usage of the SIP+FV calculator? Can you please provide pointers as to what should be entered? maybe the units.
Thanks,
-NB
NB
If you look at article , its clearly mentioned how to use it , also see the example just below the calculator , So if you want to invest Rs 1,000 per month for next 10 yrs and you expect 12% return in this 10 yrs , after that you want to leave that money for another 20 yrs and you expect a return of 10% in that 20% yrs , then you should fill
1) 1000 (monthly investment)
2) 10 (tenure of payment)
3) 12 (return expected in first 10 yrs)
4) 30 (total time = 10 + 20)
5) 10 (return expected in last 20 yrs)
Give me the number i will tell you answer .
Manish